Numerical method for the stability analysis of ideal MHD modes with a wide range of toroidal mode numbers in tokamaks
大域的・周辺局在理想MHDモードの線形安定性解析手法の確立とELM解析への応用
相羽 信行; 徳田 伸二; 藤田 隆明; 小関 隆久; Chu, M. S.*; Snyder, P. B.*; Wilson, H. R.*
Aiba, Nobuyuki; Tokuda, Shinji; Fujita, Takaaki; Ozeki, Takahisa; Chu, M. S.*; Snyder, P. B.*; Wilson, H. R.*
本研究では、Newcomb方程式に基づいた物理モデルと理想MHDモデルを併用した解析手法を開発し、ELM現象の実験計測結果との比較及び統合シミュレーションによるELM現象の物理解析や、ELMの発生によるダイバータへの熱負荷の影響が大きい大型トカマク装置の設計で求められるMHD安定性解析を可能にした。本稿においては、今回開発した2つの物理モデルの併用による解析手法の詳細、及び拡張されたMARG2Dコードと欧米において開発された低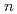 モード解析コードDCON、及び高
モード解析コードDCON、及び高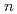 モード解析コードELITEとの国際ベンチマークテストにおいて良い一致が得られた結果を述べる。また、改修が予定されているJT-60における理想MHDモードの安定性について、特にピーリング・バルーニングモードの安定性に対する解析結果を中心に報告する。
モード解析コードELITEとの国際ベンチマークテストにおいて良い一致が得られた結果を述べる。また、改修が予定されているJT-60における理想MHDモードの安定性について、特にピーリング・バルーニングモードの安定性に対する解析結果を中心に報告する。
Numerical method for the stability analysis of ideal MHD modes is invented by using the physical model based on the two-dimensional Newcomb equation in combination with the conventional ideal MHD model. The MARG2D code built on this numerical method realizes to analyze the stability of wide 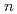 range of ideal MHD modes. The validity of MARG2D has been confirmed with the benchmarking test with the DCON code by identifying the stability boundary of low-
range of ideal MHD modes. The validity of MARG2D has been confirmed with the benchmarking test with the DCON code by identifying the stability boundary of low-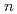 modes, and that with the ELITE code by comparing the growth rates of intermediate to high
modes, and that with the ELITE code by comparing the growth rates of intermediate to high 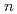 modes. With the MARG2D code, the edge stability of JT-60SA, the complementally experiment of ITER, is investigated.
modes. With the MARG2D code, the edge stability of JT-60SA, the complementally experiment of ITER, is investigated.