Phase-field model for crystallization in alkali disilicate glasses; Li O-2SiO
O-2SiO , Na
, Na O-2SiO
O-2SiO and K
and K O-2SiO
O-2SiO
二酸化アルカリケイ酸ガラスにおける結晶化のフェーズフィールドモデル; Li O-2SiO
O-2SiO , Na
, Na O-2SiO
O-2SiO 及びK
及びK O-2SiO
O-2SiO
河口 宗道 

 ; 宇埜 正美*
; 宇埜 正美*
Kawaguchi, Munemichi; Uno, Masayoshi*
本研究では、移動度係数(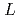 )を新しく定義することで、溶融酸化物系におけるフェーズフィールド法(PFM)の技術を開発した。一定の移動係数
)を新しく定義することで、溶融酸化物系におけるフェーズフィールド法(PFM)の技術を開発した。一定の移動係数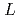 を用いたPFM計算から得られた結晶成長速度(
を用いたPFM計算から得られた結晶成長速度(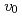 )は、normal growthモデルの熱力学的推進力と同程度であった。また
)は、normal growthモデルの熱力学的推進力と同程度であった。また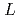 の温度依存性は、実験から得られた結晶成長速度と
の温度依存性は、実験から得られた結晶成長速度と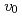 から決定し、その決定した
から決定し、その決定した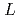 を使って、二酸化アルカリケイ酸ガラスのLi
を使って、二酸化アルカリケイ酸ガラスのLi O-2SiO
O-2SiO , Na
, Na O-2SiO
O-2SiO , K
, K O-2SiO
O-2SiO の結晶成長速度(
の結晶成長速度(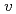 )をシミュレーションした。
)をシミュレーションした。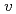 の温度依存性は定性的および定量的に非常に良く一致したため、本PFM計算は
の温度依存性は定性的および定量的に非常に良く一致したため、本PFM計算は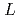 の有効性を実証した。特に、PFM計算によって得られた
の有効性を実証した。特に、PFM計算によって得られた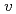 は、融点(
は、融点( )で増大し、
)で増大し、 -100Kでピークを示した。さらなる温度の下降では、
-100Kでピークを示した。さらなる温度の下降では、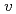 は明確に0ms
は明確に0ms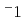 に近づくことが分かった。この振舞いは、界面のジャンプ過程を表現する
に近づくことが分かった。この振舞いは、界面のジャンプ過程を表現する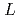 によって
によって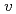 が制限されているためである。
が制限されているためである。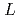 のパラメータ
のパラメータ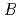 の感度についてもPFM計算を行い、
の感度についてもPFM計算を行い、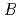 が
が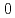 から
から まで増加すると、
まで増加すると、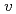 のピークはより急峻に、ピーク温度は高温側にシフトすることが分かった。アルカリ金属の原子番号が増加するにつれてイオンポテンシャルは減少するので、
のピークはより急峻に、ピーク温度は高温側にシフトすることが分かった。アルカリ金属の原子番号が増加するにつれてイオンポテンシャルは減少するので、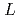 のパラメータ
のパラメータ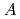 と
と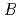 は、それぞれ指数関数的に増加、直線的に減少することになったと考えられる。本計算により
は、それぞれ指数関数的に増加、直線的に減少することになったと考えられる。本計算により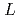 の
の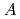 と
と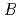 は互いに密接な関係であることが分かった。
は互いに密接な関係であることが分かった。
This study developed phase-field method (PFM) technique in oxide melt system by using a new mobility coefficient (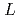 ). The crystal growth rates (
). The crystal growth rates (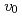 ) obtained by the PFM calculation with the constant
) obtained by the PFM calculation with the constant 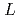 were comparable to the thermodynamic driving force in normal growth model. The temperature dependence of the
were comparable to the thermodynamic driving force in normal growth model. The temperature dependence of the 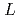 was determined from the experimental crystal growth rates and the
was determined from the experimental crystal growth rates and the 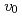 . Using the determined
. Using the determined 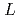 , the crystal growth rates (
, the crystal growth rates (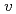 ) in alkali disilicate glasses, Li
) in alkali disilicate glasses, Li O-2SiO
O-2SiO , Na
, Na O-2SiO
O-2SiO and K
and K O-2SiO
O-2SiO were simulated. The temperature dependence of the
were simulated. The temperature dependence of the 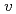 was qualitatively and quantitatively so similar that the PFM calculation results demonstrated the validity of the
was qualitatively and quantitatively so similar that the PFM calculation results demonstrated the validity of the 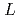 . Especially, the
. Especially, the 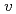 obtained by the PFM calculation appeared the rapid increase just below the thermodynamic melting point (
obtained by the PFM calculation appeared the rapid increase just below the thermodynamic melting point ( ) and the steep peak at around
) and the steep peak at around  -100 K. Additionally, as the temperature decreased, the
-100 K. Additionally, as the temperature decreased, the 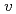 apparently approached zero ms
apparently approached zero ms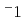 , which is limited by the
, which is limited by the 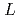 representing the interface jump process. Furthermore, we implemented the PFM calculation for the variation of the parameter
representing the interface jump process. Furthermore, we implemented the PFM calculation for the variation of the parameter 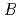 in the
in the 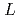 . As the
. As the 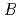 increased from zero to two, the peak of the
increased from zero to two, the peak of the 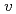 became steeper and the peak temperature of the
became steeper and the peak temperature of the 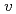 shifted to the high temperature side. The parameters
shifted to the high temperature side. The parameters 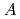 and
and 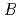 in the
in the 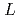 increased exponentially and decreased linearly as the atomic number of the alkali metal increased due to the ionic potential, respectively. This calculation revealed that the
increased exponentially and decreased linearly as the atomic number of the alkali metal increased due to the ionic potential, respectively. This calculation revealed that the 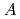 and
and 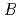 in the
in the 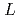 were close and reasonable for each other.
were close and reasonable for each other.